BIREP — Representations of finite dimensional algebras at Bielefeld
BIREP header picture 1
BIREP header picture 2
 

Lie Algebras

Proseminar in Winter Semester 2022/3 in English
Professor: Prof. Dr. William Crawley-Boevey


Entry in ekvv

Handout

Meetings

Fri 10:15-11:45. Organisational meeting 10:15 on 15.07.22. The organisational meeting has now passed, but there is still space for other students - please come to the first meeting on Friday 14.10.22.

Contents

A Lie algebra is a vector space \(L\) together with a bilinear operation \(L\times L\to L\) called the Lie bracket, denoted \([x,y]\) for \(x,y\in L\). The Lie bracket is not assumed to be associative or commutative; instead it should be alternating, meaning that \( [x,x] = 0\) for all \(x\in L\) and satisfy the Jacobi identity \[ [[x,[y,z]] + [y,[z,x]] + [z,[x,y]] = 0\] for all \( x,y,z\in L\). An example is given by the Lie algebra \(\mathfrak{sl}(2,\mathbb{C})\) of \(2\times 2\) matrices of trace zero over the complex numbers with the Lie bracket defined by \([x,y] = xy-yx\).

Lie algebras are closely related to Lie groups, which are groups that are also smooth manifolds, for example the general linear group, or the orthogonal group. Lie algebras appear as 'infinitessimal symmetries' of spaces, which is especially important in physics. The aim in this proseminar is to give an easy introduction to Lie algebras, hopefully getting as far as the classification of complex simple Lie algebras in terms of Dynkin diagrams

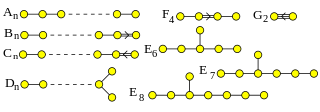
Dynkin diagrams
(source: Wikipedia)

Literature:

List of Lectures

  1. Introduction: definitions, examples, subalgebras, ideals, homomorphisms, structure constants [EW Chapter 1], 14.10.22. (JK).
  2. Ideals and homomorphism, quotient algebras, isomorphism theorems [EW Chapter 2], 21.10.22. (AW).
  3. Low-dimensional Lie algebras: Lie algebras of dimension up to 3 [EW Chapter 3], 28.10.22. (KN).
  4. Solvable and nilpotent Lie algebras [EW Chapter 4], 04.11.22. (SD).
  5. Subalgebras of \(\mathfrak{gl}(V)\) [EW Chapter 5]. (PK).
  6. Engel's Theorem and Lie's Theorem [EW Chapter 6].
  7. Representation Theory [EW Chapter 7. Not yet assigned].
  8. Representations of \(\mathfrak{sl}(2,\mathbb{C})\) [EW Chapter 8].
  9. Cartan's Criteria [EW Chapter 9].
  10. Root space decomposition [EW Chapter 10].
  11. Root systems [EW Chapter 11].
  12. The classical Lie algebras [EW Chapter 12].
  13. The classification of Root Systems [EW Chapter 13].
  14. Simple Lie algebras [EW Chapter 14].
  15. Irreducible representations of a semisimple Lie algebras [EW Section 15.1].

Note: if there is not enough suggested material to fill a talk, then students should explain some exercises from the relevant chapter of [EW] or use the other references.