Section 1.1 of
- Fomin and Reading: Root systems and generalized associahedra (Geometric combinatorics, 63-131, IAS/Park City Math. Ser., 13, Amer. Math. Soc., Providence, RI, 2007)
As Fomin points out, it would be a stretch to say that Gauss discovered cluster algebras: the pentagon equation, in its algebraic form, was found by W. Spence in 1809. In the context of Pentagramma Mirificum, the realization that the 5-cycle represents a nontrivial algebraic identity seems to appear first in print by Arthur Cayley; see [A. Cayley, On Gauss's Pentagramma Mirificum, Philosophical Magazine, vol. XLIL (1871), 311-312; The collected mathematical papers of Arthur Cayley. Vol. 7].
In the 20th century, the topic was popularized by H. S. M. Coxeter; see [Kaleidoscopes, pages 85-103] and [Non-Euclidean geometry, Section 12.7].
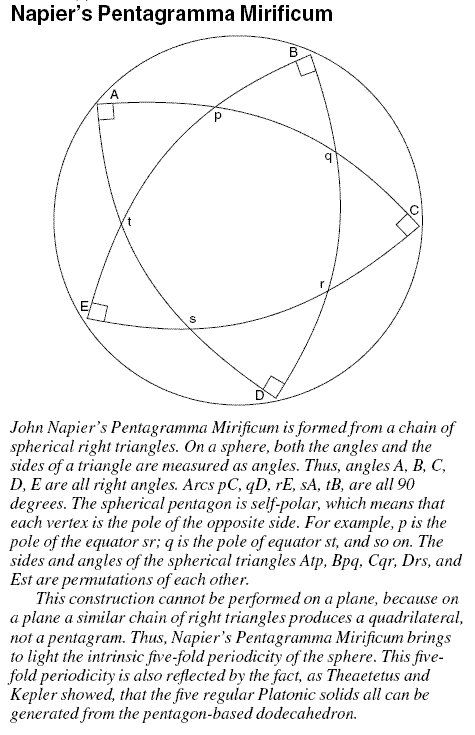
From: Bruce Director: From Plato.s Theaetetus to Gauss.s Pentagramma Mirificum: A Fight for Truth.